.
Curve of constant width
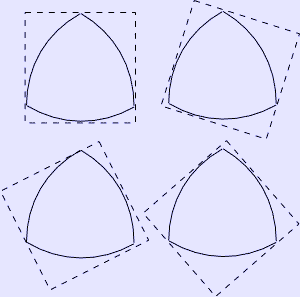
A Reuleaux triangle is a curve of constant width. Opposite sides on each square are parallel supporting lines, since each touches the curve but does not intersect the interior. The fact that the square can be rotated whilst still just intersecting the boundary shows that width (separation between parallel supporting lines) is constant in all directions.
In geometry, a curve of constant width is a convex planar shape whose width, measured by the distance between two opposite parallel lines touching its boundary, is the same regardless of the direction of those two parallel lines. One defines the width of the curve in a given direction to be the perpendicular distance between the parallels perpendicular to that direction.
More generally, any compact convex planar body D has one pair of parallel supporting lines in a given direction. A supporting line is a line that intersects the boundary of D but not the interior of D. One defines the width of the body as before. If the width of D if the same in all directions, then one says that the body is of constant width and calls its boundary a curve of constant width, and the planar body itself is referred to as an orbiform.
Curves of constant width can be rotated between parallel line segments. To see this, simply note that one can rotate parallel line segments (supporting lines) around curves of constant width by definition. Consequently, a curve of constant width can be rotated in a square.
The circle is obviously a curve of constant width. A nontrivial example is the Reuleaux triangle. To construct this, take an equilateral triangle ABC and draw the arc BC on the circle centered at A, the arc CA on the circle centered at B, and the arc AB on the circle centered at C. The resulting figure is of constant width.
A basic result on curves of constant width is Barbier's theorem, which asserts that the perimeter of any curve of constant width is equal to the width (diameter) multiplied by π. A simple example of this would be a circle with width (diameter) d having a perimeter of πd.
By the isoperimetric inequality and Barbier's theorem, the circle has the maximum area of any curve of given constant width. The Blaschke-Lebesgue theorem says that the Reuleaux triangle had the least area of any curve of given constant width.
Δ curves, or curves which can be rotated in the equilateral triangle, have many similar properties to curves of constant width. The generalization of the definition of bodies of constant width to convex bodies in R³ and their boundaries leads to the concept of surface of constant width (in the case of a Reuleaux triangle, this does not lead to a Reuleaux tetrahedron, but to a Meissner body). There is also the concept of space curves of constant width, which are space curves whose normal planes intersect them at exactly two points and are normal to them at both points.
Famous examples of a curve of constant width are the British 20p and 50p coins. Their heptagonal shape with curved sides means that the currency detector in an automated coin machine will always measure the correct diameter through the middle, no matter which angle it takes its measurement from.
A curve of constant width is also used in the Wankel engine, which uses a Reuleaux triangle with slightly flattened corners as a rotor within an epitrochoidal combustion chamber. The property of constant width allows the rotor to pass eccentrically through the chamber, maintaining seals against the walls while compressing and expanding volumes of a fluid. In practice, the seals have proven very difficult to maintain.
Links
* Star Construction of Shapes of Constant Width at cut-the-knot
Undergraduate Texts in Mathematics
Graduate Studies in Mathematics
Retrieved from "http://en.wikipedia.org/"
All text is available under the terms of the GNU Free Documentation License

