.
Poincaré disk model
In geometry, the Poincaré disk model, also called the conformal disk model, is a model of n-dimensional hyperbolic geometry in which the points of the geometry are in an n-dimensional disk, or unit ball, and the straight lines of the hyperbolic geometry are segments of circles contained in the disk orthogonal to the boundary of the disk, or else diameters of the disk. Along with the Klein model and the Poincaré half-space model, it was proposed by Eugenio Beltrami who used these models to show hyperbolic geometry was equiconsistent with Euclidean geometry.
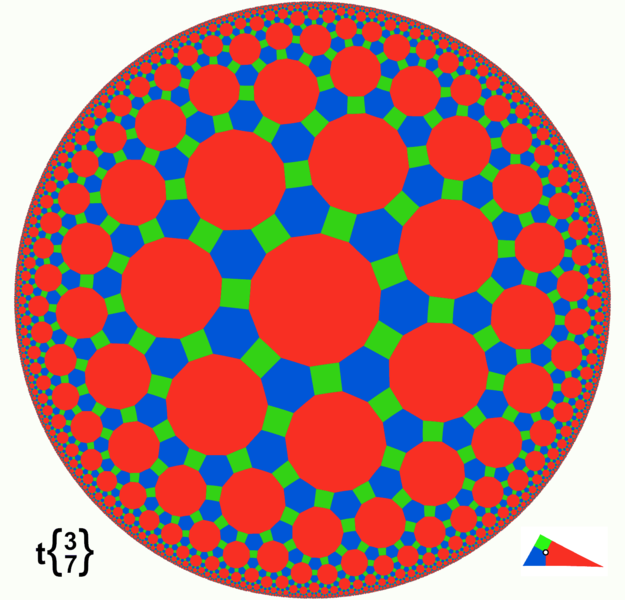
Poincaré disc model of great rhombitruncated {3,7} tiling.
Metric
If u and v are two vectors in real n-dimensional vector space Rn with the usual Euclidean norm, both of which have norm less than 1, then we may define an isometric invariant by
\( \delta (u, v) = 2 \frac{\lVert u-v \rVert^2}{(1-\lVert u \rVert^2)(1-\lVert v \rVert^2)},\, \)
where \( \lVert \cdot \rVert \) denotes the usual Euclidean norm. Then the distance function is
\( d(u, v) = \operatorname{arccosh} (1+\delta (u,v)).\, \)
Such a distance function is defined for any two vectors of norm less than one, and makes the set of such vectors into a metric space which is a model of hyperbolic space of constant curvature −1. The model has the conformal property that the angle between two intersecting curves in hyperbolic space is the same as the angle in the model.
The associated metric tensor of the Poincaré disk model is given by
\( ds^2 = 4 \frac{\sum_i dx_i^2}{(1-\sum_i x_i^2)^2} \)
where the xi are the Cartesian coordinates of the ambient Euclidean space. The geodesics of the disk model are circles perpendicular to the boundary sphere Sn−1.
Relation to the hyperboloid model
Red circular arc is geodesic in Poincaré disk model; it projects to the brown geodesic on the green hyperboloid.
The Poincaré disk model, as well as the Klein model, are related to the hyperboloid model projectively. If we have a point [t, x1, ..., xn] on the upper sheet of the hyperboloid of the hyperboloid model, thereby defining a point in the hyperboloid model, we may project it onto the hypersurface t = 0 by intersecting it with a line drawn through [−1, 0, ..., 0]. The result is the corresponding point of the Poincaré disk model.
For Cartesian coordinates (t, xi) on the hyperboloid and (yi) on the plane, the conversion formulae are:
\( y_i = \frac{x_i}{1 + t} \)
\( (t, x_i) = \frac {\left( 1+\sum{y_i^2},\, 2 y_i \right)} {1-\sum{y_i^2}} \)
Compare the formulae for stereographic projection between a sphere and a plane.
Analytic geometry constructions in the hyperbolic plane
A basic construction of analytic geometry is to find a line through two given points. In the Poincaré disk model, lines in the plane are defined by portions of circles having equations of the form
\( x^2 + y^2 + a x + b y + 1 = 0,\, \)
which is the general form of a circle orthogonal to the unit circle, or else by diameters. Given two points u and v in the disk which do not lie on a diameter, we can solve for the circle of this form passing through both points, and obtain
\( \begin{align} & {} \quad x^2 + y^2 + \frac{u_2(v_1^2+v_2^2)-v_2(u_1^2+u_2^2)+u_2-v_2}{u_1v_2-u_2v_1}x \\[8pt] & {} + \frac{v_1(u_1^2+u_2^2)-u_1(v_1^2+v_2^2)+v_1-u_1}{u_1v_2-u_2v_1}y + 1 = 0. \end{align} \)
If the points u and v are points on the boundary of the disk not lying at the endpoints of a diameter, the above simplifies to
\( x^2+y^2+\frac{2(u_2-v_2)}{u_1v_2-u_2v_1}x - \frac{2(u_1-v_1)}{u_1v_2-u_2v_1}y + 1 = 0. \)
Angles
We may compute the angle between the circular arc whose endpoints (ideal points) are given by unit vectors u and v, and the arc whose endpoints are s and t, by means of a formula. Since the ideal points are the same in the Klein model and the Poincaré disk model, the formulas are identical for each model.
If both models' lines are diameters, so that v = −u and t = −s, then we are merely finding the angle between two unit vectors, and the formula for the angle θ is
\( \cos(\theta) = u \cdot s.\, \)
If v = −u but not t = −s, the formula becomes, in terms of the wedge product,
\( \cos^2(\theta) = \frac{P^2}{QR}, \)
where
\( P = u \cdot (s-t),\, \)
\( Q = u \cdot u,\, \)
\( R = (s-t) \cdot (s-t) - (s \wedge t) \cdot (s \wedge t).\, \)
If both chords are not diameters, the general formula obtains
\( \cos^2(\theta) = \frac{P^2}{QR}, \)
where
\( P = (u-v) \cdot (s-t) - (u \wedge v) \cdot (s \wedge t),\, \)
\( Q = (u-v) \cdot (u-v) - (u \wedge v) \cdot (u \wedge v),\, \)
\( R = (s-t) \cdot (s-t) - (s \wedge t) \cdot (s \wedge t).\, \)
Using the Binet–Cauchy identity and the fact that these are unit vectors we may rewrite the above expressions purely in terms of the dot product, as
\( P = (u-v) \cdot (s-t) + (u \cdot t)(v \cdot s) - (u \cdot s)(v \cdot t),\, \)
\( Q = (1 - u \cdot v)^2,\, \)
\( R = (1 - s \cdot t)^2.\, \)
Artistic realizations
The M.C. Escher print Circle Limit IV is an artistic visualization of the Poincaré disk.
See also
Hyperbolic geometry
Klein model
Poincaré half-plane model
Poincaré metric
Pseudosphere
Hyperboloid model
Inversive geometry
Uniform tilings in hyperbolic plane
References
James W. Anderson, Hyperbolic Geometry, second edition, Springer, 2005
Eugenio Beltrami, Teoria fondamentale degli spazii di curvatura costante, Annali. di Mat., ser II 2 (1868), 232-255
Saul Stahl, The Poincaré Half-Plane, Jones and Bartlett, 1993
Undergraduate Texts in Mathematics
Graduate Studies in Mathematics
Retrieved from "http://en.wikipedia.org/"
All text is available under the terms of the GNU Free Documentation License

