Niven's constant

In number theory, Niven's constant, named after Ivan Niven, is the largest exponent appearing in the prime factorization of any natural number n "on average". More precisely, if we define H(1) = 1 and H(n) = the largest exponent appearing in the unique prime factorization of a natural number n > 1, then Niven's constant is given by

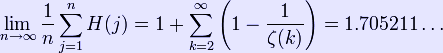
where ζ(k) is the value of the Riemann zeta function at the point k (Niven, 1969).

In the same paper Niven also proved that

where h(1) = 1, h(n) = the smallest exponent appearing in the unique prime factorization of each natural number n > 1, o is little o notation, and the constant c is given by

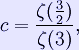
and consequently that

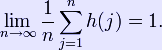
References

* Niven, Ivan M. (August 1969). "Averages of Exponents in Factoring Integers". Proceedings of the American Mathematical Society 22 (2): 356–360. doi:10.2307/2037055. http://links.jstor.org/sici?sici=0002-9939(196908)22%3A2%3C356%3AAOEIFI%3E2.0.CO%3B2-1. Retrieved 2007-03-08.
* Steven R. Finch, Mathematical Constants (Encyclopedia of Mathematics and its Applications), Cambridge University Press, 2003

External links

* Weisstein, Eric W., "Niven's Constant" from MathWorld.
* Sloane's A033150 . The On-Line Encyclopedia of Integer Sequences (external link). AT&T Labs Research.