- Eric W. Weisstein, Hypercube at MathWorld.
- Olshevsky, George, Measure polytope at Glossary for Hyperspace.
- Multi-dimensional Glossary: hypercube Garrett Jones
.
Enneract
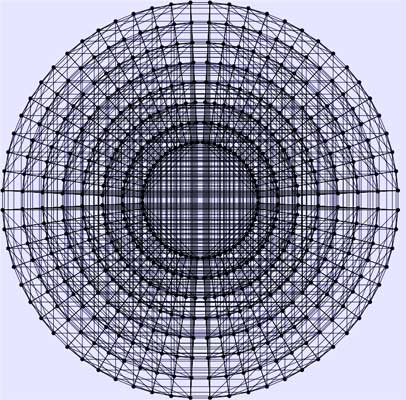
Vertex-edge graph. (*)
| Enneract 9-cube |
|
|---|---|
| Type | Regular 9-polytope |
| Family | hypercube |
| Schläfli symbol | {4,3,3,3,3,3,3,3} |
| Coxeter-Dynkin diagram | |
| 8-faces | 18 octeracts |
| 7-faces | 144 hepteracts |
| 6-faces | 672 hexeracts |
| 5-faces | 2016 penteracts |
| 4-faces | 4032 tesseracts |
| Cells | 5376 cubes |
| Faces | 4608 squares |
| Edges | 2304 |
| Vertices | 512 |
| Vertex figure | 8-simplex |
| Symmetry group | B9, [3,3,3,3,3,3,3,4] |
| Dual | Enneacross |
| Properties | convex |
An enneract is a nine-dimensional hypercube with 512 vertices, 2304 edges, 4608 square faces, 5376 cubic cells, 4032 tesseract 4-faces, 2016 penteract 5-faces, 672 hexeract 6-faces, 144 hepteract 7-faces, and 18 octeract 8-faces.
The name enneract is derived from combining the name tesseract (the 4-cube) with enne for nine (dimensions) in Greek.
It can also be called a regular octadeca-9-tope or octadecayotton, being made of 18 regular facets.
It is a part of an infinite family of polytopes, called hypercubes. The dual of an enneract can be called a enneacross, and is a part of the infinite family of cross-polytopes.
Cartesian coordinates
Cartesian coordinates for the vertices of a penteract centered at the origin and edge length 2 are
(±1,±1,±1,±1,±1,±1,±1,±1,±1)
while the interior of the same consists of all points (x0, x1, x2, x3, x4, x5, x6, x7, x8) with −1 < xi < 1.
Projections
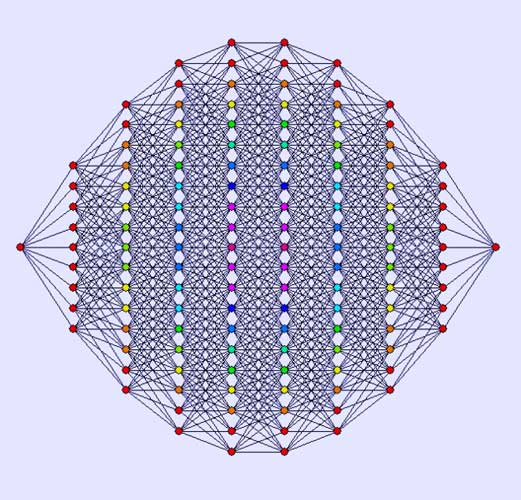
An orthogonal projection viewed along the axes of two opposite vertices and the average plane of one edge path between.
Derived polytopes
Applying an alternation operation, deleting alternating vertices of the enneract, creates another uniform polytope, called a demienneract, (part of an infinite family called demihypercubes), which has 18 demiocteractic and 256 enneazettonic facets.
See also
* Hypercubes family
o square - {4}
o Cube - {4,3}
o Tesseract - {4,3,3}
o Penteract - {4,3,3,3}
o Hexeract - {4,3,3,3,3}
o Hepteract - {4,3,3,3,3,3}
o Octeract - {4,3,3,3,3,3,3}
o ...
References
* Coxeter, H.S.M. Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8 p.296, Table I (iii): Regular Polytopes, three regular polytopes in n-dimensions (n>=5)
Links
Undergraduate Texts in Mathematics
Graduate Studies in Mathematics
Retrieved from "http://en.wikipedia.org/"
All text is available under the terms of the GNU Free Documentation License

