.
Hyperbolic triangle
In mathematics, the term hyperbolic triangle has more than one meaning.
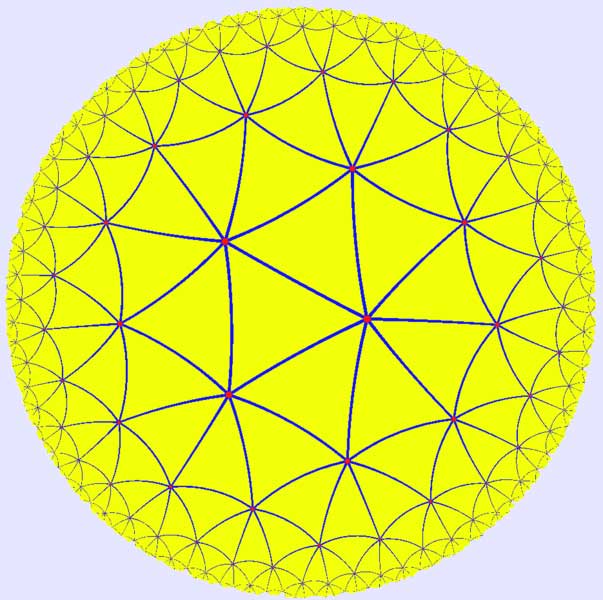
A tiling of the hyperbolic plane with hyperbolic triangles – the order-7 triangular tiling. (*)
Hyperbolic geometry
In hyperbolic geometry, a hyperbolic triangle is a figure in the hyperbolic plane, analogous to a triangle in Euclidean geometry, consisting of three sides and three angles. The relations among the angles and sides are analogous to those of spherical trigonometry; they are most conveniently stated if the lengths are measured in terms of a special unit of length analogous to a radian. In terms of the Gaussian curvature K of the plane this unit is given by
\( R=\frac{1}{\sqrt{-K}}. \)
In all the trig formulas stated below the sides a, b, and c must be measured in this unit. In a hyperbolic triangle the sum of the angles A, B, C (respectively opposite to the side with the corresponding letter) is strictly less than a straight angle. The difference is often called the defect of the triangle. The area of a hyperbolic triangle is equal to its defect multiplied by the square of R:
\( (\pi-A-B-C) R^2{}{}.\! \)
The corresponding theorem in spherical geometry is Girard's theorem first proven by Johann Heinrich Lambert.
Right triangles
If C is a right angle then:
The sine of angle A is the ratio of the hyperbolic sine of the side opposite the angle to the hyperbolic sine of the hypotenuse.
\( \sin A=\frac{\textrm{sinh(opposite)}}{\textrm{sinh(hypotenuse)}}=\frac{\sinh a}{\,\sinh c\,}.\, \)
The cosine of angle A is the ratio of the hyperbolic tangent of the adjacent leg to the hyperbolic tangent of the hypotenuse.
\( \cos A=\frac{\textrm{tanh(adjacent)}}{\textrm{tanh(hypotenuse)}}=\frac{\tanh b}{\,\tanh c\,}.\, \)
The tangent of angle A is the ratio of the hyperbolic tangent of the opposite leg to the hyperbolic sine of the adjacent leg.
\( \tan A=\frac{\textrm{tanh(opposite)}}{\textrm{sinh(adjacent)}}=\frac{\tanh a}{\,\sinh b\,}.\, \)
The hyperbolic sine, cosine, and tangent are hyperbolic functions which are analogous to the standard trigonometric functions.
Oblique triangles
Whether C is a right angle or not, the following relationships hold.
There is a law of cosines:
\( \cosh c=\cosh a\cosh b-\sinh a\sinh b \cos C,\, \)
its dual:
\( \cos C= -\cos A\cos B+\sin A\sin B \cosh c,\, \)
a law of sines:
\( \frac{\sin A}{\sinh a} = \frac{\sin B}{\sinh b} = \frac{\sin C}{\sinh c}, \)
and a four-parts formula:
\( \cos C\cosh a=\sinh a\coth b-\sin C\cot B.\, \)
Ideal triangles
If a pair of sides is asymptotic they may be said to form an angle of zero. In projective geometry, they meet at an ideal vertex on the circle at infinity. If all three are vertices are ideal, then the resulting figure is called an ideal triangle. An ideal hyperbolic triangle has an angle sum of 0°, a property it has in common with the triangular area in the Euclidean plane bounded by three tangent circles.
Euclidean geometry
In the foundations of the hyperbolic functions sinh, cosh and tanh, a hyperbolic triangle is a right triangle in the first quadrant of the Cartesian plane
\( \{(x,y):x,y \in \mathbb R\}, \)
with one vertex at the origin, base on the diagonal ray y = x, and third vertex on the hyperbola
\( xy=1.\, \)
The length of the base of such a triangle is
\( \sqrt 2 \cosh a,\, \)
and the altitude is
\( \sqrt 2 \sinh a,\, \)
where a is the appropriate hyperbolic angle.
See also
Hyperbolic law of cosines
Pair of pants
Triangle group
References
Augustus De Morgan (1849) Trigonometry and Double Algebra, Chapter VI: "On the connection of common and hyperbolic trigonometry".
Stothers, Wilson (2000), Hyperbolic geometry, University of Glasgow, interactive instructional website.
Svetlana Katok, Fuchsian Groups (1992), University of Chicago Press, Chicago ISBN 0-226-42583-5 (Provides a brief but simple, easily readable review in chapter 1.)
Undergraduate Texts in Mathematics
Graduate Studies in Mathematics
Retrieved from "http://en.wikipedia.org/"
All text is available under the terms of the GNU Free Documentation License

