General relativity or the general theory of relativity is the geometric theory of gravitation published by Albert Einstein in 1915/16. It unifies special relativity and Newton's law of universal gravitation, resulting in a description of gravity as a property of the geometry of space and time. In particular, the curvature of spacetime is directly related to the four-momentum (mass-energy and linear momentum) of whatever matter and radiation are present. The relation is specified by the Einstein field equations, a system of partial differential equations.

General relativity predicts novel effects relating to the passage of time, the geometry of space, the motion of bodies in free fall, and the propagation of light. Examples are gravitational time dilation, the gravitational redshift of light, and the gravitational time delay; the theory's predictions have been confirmed in numerous observations and experiments. Although not the only relativistic theory of gravity, general relativity is the simplest such theory that is consistent with the experimental data. Still, a number of open questions remain, the most fundamental being how general relativity can be reconciled with the laws of quantum physics to produce a complete and self-consistent theory of quantum gravity.

Einstein's theory has important astrophysical applications. It points towards the existence of black holes—regions of space in which space and time are distorted in such a way that nothing, not even light, can escape—as an end-state for massive stars. There is evidence that, indeed, such stellar black holes as well as more massive varieties of black hole are responsible for the intense radiation emitted by certain types of astronomical objects (such as active galactic nuclei or microquasars). The bending of light by gravity can lead to the curious phenomenon of gravitational lensing, where multiple images of the same distant astronomical object are visible in the sky. General relativity also predicts the existence of gravitational waves, which have since been measured indirectly; a direct measurement is the aim of projects such as LIGO. In addition, general relativity is the basis of current cosmological models of an expanding universe.

History

Main articles: History of general relativity, Golden age of general relativity, and Classical theories of gravitation

Soon after publishing his theory of special relativity in 1905, Einstein started to think about how to incorporate gravity into his new relativistic framework. In 1907, beginning with a simple thought experiment involving an observer in free fall, he embarked on what would be an eight-year search for a relativistic theory of gravity. After numerous detours and false starts, his work culminated in the November, 1915 presentation to the Prussian Academy of Science of what are now known as the Einstein field equations. These equations specify how the geometry of space and time is influenced by whatever matter is present, and form the core of Einstein's general theory of relativity.[1]

In his own derivation of his theory's predictions, Einstein had worked with approximation methods. But as early as 1916, the astrophysicist Schwarzschild found the first non-trivial exact solution to the Einstein field equations. It is nowadays known under his name, and laid the groundwork for the description of the final stages of gravitational collapse, and the objects which eventually became known as a black holes. The same year saw the first steps of generalization to electrically charged objects that would result in the Reissner-Nordström solution, now associated with electrically charged black holes.[2] In 1917, Einstein applied his theory to the universe as a whole, initiating the field of relativistic cosmology. In line with contemporary thinking, he was set on describing a static universe and, to achieve that goal, added a new parameter to his original field equations, the cosmological constant.[3] When, in 1929, the work of Hubble and others made it clear that our universe is indeed expanding, and thus better described by expanding cosmological solutions found by Friedmann in 1922, Lemaître formulated the earliest version of the big bang models.[4]

During all that time, general relativity remained something of a curiosity among physical theories. There was evidence that it was to be preferred to Newtonian gravity: Einstein himself had shown in 1915 how his theory effortlessly explained the anomalous perihelion advance of the planet Mercury,[5] and a 1919 expedition led by Eddington had announced confirmation of general relativity's prediction for the deflection of starlight by the Sun[6] (instantly catapulting Einstein to world fame[7]). Yet it was only with the developments between approximately 1960 and 1975, now known as the Golden age of general relativity, that the theory entered the mainstream of theoretical physics and astrophysics. Physicists began to understand the concept of a black hole, and to identify these objects' astrophysical manifestation as quasars.[8] Ever more precise solar system tests confirmed the theory's predictive power,[9] and relativistic cosmology, too, became amenable to direct observational tests.[10]

From classical mechanics to general relativity

General relativity is best understood by examining its similarities with and departures from classical physics. The first step is the realization that classical mechanics and Newton's law of gravity admit of a geometric description. Combining this description with the laws of special relativity, one can heuristically derive general relativity.[11]

Geometry of Newtonian gravity

At the base of classical mechanics is the notion that a body's motion can be described as a combination of free (or inertial) motion, and deviations from this free motion. Such deviations are caused by external forces acting on a body in accordance with Newton's second law of motion, which states that the force acting on a body is equal to that body's (inertial) mass times its acceleration.[12] The preferred inertial motions are related to the geometry of space and time: in the standard reference frames of classical mechanics, objects in free motion move along straight lines at constant speed. In modern parlance, their paths are geodesics, straight world lines in spacetime.[13]

Conversely, one might expect that inertial motions, once identified by observing the actual motions of bodies and making allowances for the external forces (such as electromagnetism or friction), can be used to define the geometry of space, as well as a time coordinate. However, there is an ambiguity once gravity comes into play. According to Newton's law of gravity, and independently verified by experiments such as that of Eötvös and its successors, there is a universality of free fall (also known as the weak equivalence principle, or the universal equality of inertial and passive-gravitational mass): the trajectory of a test body in free fall depends only on its position and initial speed, but not on any of its material properties.[14] A simplified version of this is embodied in Einstein's elevator experiment, illustrated in the figure on the right: for an observer in a small enclosed room, it is impossible to decide, by mapping the trajectory of bodies such as a dropped ball, whether the room is at rest in a gravitational field, or in free space aboard an accelerated rocket.[15]

Given the universality of free fall, there is no observable distinction between inertial motion and motion under the influence of the gravitational force. This suggests the definition of a new class of inertial motion, namely that of objects in free fall under the influence of gravity. This new class of preferred motions, too, defines a geometry of space and time—in mathematical terms, it is the geodesic motion associated with a specific connection which depends on the gradient of the gravitational potential. Space, in this construction, still has the ordinary Euclidean geometry. However, as can be shown using simple thought experiments following the free-fall trajectories of different test particles, the result of transporting spacetime vectors that can denote a particle's velocity (time-like vectors) will vary with the particle's trajectory; in mathematical terms: the Newtonian connection is not integrable. From this, one can deduce that spacetime is curved. The result is a geometric formulation of Newtonian gravity using only covariant concepts, in other words: a description which is valid in any desired coordinate system.[16] In this geometric description, tidal effects—the relative acceleration of bodies in free fall—are related to the derivative of the connection, showing how the modified geometry is caused by the presence of mass.[17]

Relativistic generalization

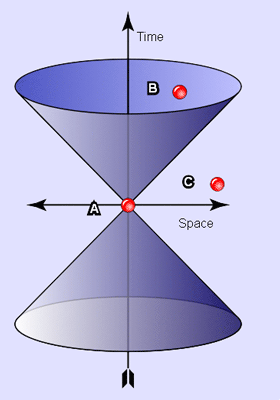
Light cone

As intriguing as geometric Newtonian gravity may be, its basis, classical mechanics, is merely a limiting case of (special) relativistic mechanics.[18] In the language of symmetry: where gravity can be neglected, physics is Lorentz invariant as in special relativity rather than Galilei invariant as in classical mechanics. (The defining symmetry of special relativity is the Poincaré group which also includes translations and rotations.) The differences between the two become significant when we are dealing with speeds approaching the speed of light, and with high-energy phenomena.[19]

With Lorentz symmetry, additional structures comes into play. They are defined by the set of light cones (see the image on the left). The light-cones define a causal structure: for each event A, there is a set of events that can, in principle, either influence or be influenced by A via signals or interactions that do not need to travel faster than light (such as event B in the image), and a set of events for which such an influence is impossible (such as event C in the image). These sets are observer-independent.[20] But the light-cones, in conjunction with the world-lines of freely falling particles, contain even more information: they can be used to reconstruct the space-time's semi-Riemannian metric, at least up to a positive scalar factor. In mathematical terms, this defines a conformal structure.[21]

Special relativity is defined in the absence of gravity, so for practical applications, it is a suitable model whenever gravity can be neglected. Bringing gravity into play, and assuming the universality of free fall, an analogous reasoning as in the previous section applies: there are no global inertial frames. Instead there are approximate inertial frames moving alongside freely falling particles. Translated into the language of spacetime: the straight time-like lines that define a gravity-free inertial frame are deformed to lines that are curved relative to each other, suggesting that the inclusion of gravity necessitates a change in spacetime geometry.[22]

A priori, it is not clear whether the new local frames in free fall are indeed those in which the laws of special relativity hold—that theory is based on the propagation of light, and thus on electromagnetism, which could have a different set of preferred frames. But using different assumptions about the special-relativistic frames (such as their being earth-fixed, or in free fall), one can derive different predictions for the gravitational redshift, that is, the way in which the frequency of light shifts as the light propagates through a gravitational field (cf. below). The actual measurements show that free-falling frames are the ones in which light propagates as it does in special relativity.[23] The generalization of this statement, namely that the laws of special relativity hold, to good approximation, in freely falling (and non-rotating) reference frames, is known as the Einstein equivalence principle, a crucial guiding principle for generalizing special-relativistic physics to include gravity.[24]

The same experimental data shows that time as measured by clocks in a gravitational field—proper time, to give the technical term—does not follow the rules of special relativity. In the language of spacetime geometry, it is not measured by the Minkowski metric. As in the Newtonian case, this is suggestive of a more general geometry. At small scales, all reference frames that are in free fall are equivalent, and approximately Minkowskian. Consequently, we are now dealing with a curved generalization of Minkowski space. The metric tensor that defines the geometry—in particular, how lengths and angles are measured—is not the Minkowski metric of special relativity, it is a generalization known as a semi- or pseudo-Riemannian metric. Furthermore, each Riemannian metric is naturally associated with one particular kind of connection, the Levi-Civita connection, and this is, in fact, the connection that satisfies the equivalence principle and makes space locally Minkowskian (that is, in suitable "locally inertial" coordinates, the metric is Minkowskian, and its derivatives and the connection coefficients vanish).[25]

Einstein's equations

Main article: Einstein field equations

Having formulated the relativistic, geometric version of the effects of gravity, the question of gravity's source remains. In Newtonian gravity, the source is mass. In special relativity, mass turns out to be part of a more general quantity called the energy-momentum tensor, which includes both energy and momentum densities as well as stress (that is, pressure and shear).[26] Using the equivalence principle, this tensor is readily generalized to curved space-time. Drawing further upon the analogy with geometric Newtonian gravity, it is natural to assume that the field equation for gravity relates this tensor and the Ricci tensor, which describes a particular class of tidal effects: the change in volume for a small cloud of test particles that are initially at rest, and then fall freely. In special relativity, conservation of energy-momentum correspond to the statement that the energy-momentum tensor is divergence-free. This formula, too, is readily generalized to curved spacetime by replacing partial derivatives with their curved-manifold counterparts, covariant derivatives. With this additional condition—the covariant divergence of the energy-momentum tensor, and hence of whatever is on the other side of the equation, is zero— the simplest set of equations are what are called Einstein's (field) equations. They equate the energy-momentum tensor and a specific divergence-free combination of the Ricci tensor and the metric, which is known as the Einstein tensor:

,

where Gab is the Einstein tensor, Tab is the energy-momentum tensor (both written in abstract index notation).[27] Matching the theory's prediction to observational results for planetary orbits (or, equivalently, assuring that the weak-gravity, low-speed limit is Newtonian mechanics), the proportionality constant can be fixed as κ = 8πG/c4, with G the gravitational constant and c the speed of light.[28]

It is worth mentioning that there are alternatives to general relativity built upon the same premises, which include additional rules and/or constraints, leading to different field equations. Examples are Brans-Dicke theory, teleparallelism, and Einstein-Cartan theory.[29]

Definition and basic applications

See also: Mathematics of general relativity and Physical theories modified by general relativity

The derivation outlined in the previous section contains all the information needed to define and characterize general relativity. Having the defined the theory, we will address a question of crucial importance in physics: how the theory can be used for model-building.

Definition and basic properties

General relativity is a metric theory of gravitation. At its core are Einstein's equations, which describe the relation between the geometry of a four-dimensional, semi-Riemannian manifold representing spacetime on the one hand, and the energy-momentum contained in that spacetime on the other.[30] Phenomena that, in classical mechanics, are ascribed to the action of the force of gravity (such as free-fall, orbital motion, and spacecraft trajectories), correspond to inertial motion within a curved geometry of spacetime in general relativity: there is no gravitational force deflecting objects from their natural, straight paths. Instead, gravity changes the properties of space and time, which changes the straightest-possible paths that objects will naturally follow.[31] The curvature is, in turn, caused by the energy-momentum of matter. Paraphrasing the relativist John Archibald Wheeler, spacetime tells matter how to move; matter tells spacetime how to curve.[32]

While general relativity replaces the scalar gravitational potential of classical physics by a symmetric rank-two tensor, the latter reduces to the former in certain limiting cases. For weak gravitational fields and slow speed relative to the speed of light, the theory's predictions converge on those of Newton's law of gravity.[33]

As it is constructed using tensors, general relativity exhibits general covariance: its laws—and further laws formulated within the general relativistic framework—take on the same form in all coordinate systems.[34] Furthermore, the theory does not contain any invariant geometric background structures. It thus satisfies a more stringent general principle of relativity, namely that the laws of physics are the same for all observers.[35] Locally, as expressed in the equivalence principle, spacetime is Minkowskian, and the laws of physics have local Lorentz invariance.[36]

Model-building

The core concept of general-relativistic model-building is that of a solution of Einstein's equations. Given both Einstein's equations and suitable equations for the properties of matter, such a solution consists of a specific semi-Riemannian manifold (usually defined by giving the metric in specific coordinates), and specific matter fields defined on that manifold. Matter and geometry must satisfy Einstein's equations, and the matter must satisfy whatever additional equations have been imposed on its properties. In short, such a solution is a model universe that satisfies the laws of general relativity, and possibly additional laws governing whatever matter might be present.[37]

Einstein's equations are non-linear partial differential equations and, as such, very difficult to solve.[38] Nevertheless, a number of exact solutions are known, although only a few of them have direct physical applications.[39] The best-known exact solutions, and also those most interesting from a physics point of view, are the Schwarzschild solution, the Reissner-Nordström solution and the Kerr metric, each corresponding to a certain type of black hole in an otherwise empty universe,[40] and the Friedmann-Lemaître-Robertson-Walker and de Sitter universes, each describing an expanding cosmos.[41] Exact solutions of great theoretical interest include the Gödel universe (which opens up the intriguing possibility of time travel in curved spacetimes), the Taub-NUT solution (a model universe that is homogeneous, but anisotropic), and Anti-de Sitter space (which has recently come to prominence in the context of what is called the Maldacena conjecture).[42]

Significant efforts are being made in the field of numerical relativity, where powerful computers are employed to find interesting numerical solutions describing, say, two black holes orbiting each other.[43] Also, there are different methods for finding approximate solutions in the context of perturbation theory. The best-known of these are linearized gravity[44] and its generalization, the Post-Newtonian expansion. The latter provides for a systematic way of describing a spacetime that contains matter which is not particularly compact, and which moves slowly compared with the speed of light. The expansion involves a series of terms. The first terms represent Newtonian gravity. Additional terms systematically represent smaller and smaller effects arising from the difference between Newton's theory and general relativity.[45] An extension of this expansion is the Parametrized Post-Newtonian (PPN) formalism, a framework for making quantitative comparisons between the predictions of general relativity and alternative theories.[46]

Consequences of Einstein's theory

General relativity has a number of physical consequence. Some follow directly from the theory's axioms, while others have become clear only in the course of the ninety years of research that followed Einstein's initial publication.

Gravitational time dilation and frequency shift

Main article: Gravitational time dilation

In any theory in which the equivalence principle holds,[47] gravity has an immediate influence on the passage of time. Light sent down into a gravity well is blueshifted, light climbing out of a gravity well, redshifted in what is known as the gravitational frequency shift. Closely related is the fact that processes near massive bodies run more slowly when compared with processes taking place further away, an effect known as gravitational time dilation.[48]

Gravitational redshift has been measured in the laboratory[49] and using astronomical observations.[50] Gravitational time dilation in the Earth's gravitational field has been measured numerous times using atomic clocks,[51] while ongoing validation is provided as a side-effect of the operation of the Global Positioning System (GPS).[52] Tests in stronger gravitational fields are provided by the observation of binary pulsars.[53] All results are in agreement with general relativity.[54] However, at the current level of accuracy, these observations cannot distinguish between general relativity and other theories in which the equivalence principle is valid.[55]

Light deflection and gravitational time delay

Main articles: Kepler problem in general relativity, Gravitational lens, and Shapiro delay

In general relativity, light follows what are called light-like or null geodesics—a generalization of the straight lines along which light travels in classical physics, and the invariance of lightspeed in special relativity.[56] As one examines suitable model spacetimes (either the exterior Schwarzschild solution or, for more than a single mass, the Post-Newtonian expansion),[57] several effects of gravity on light propagation emerge.

The best-known is the bending of light in a gravitational field: light passing a massive body is deflected towards that body. While such an effect can also be derived by extending the universality of free fall to light,[58] the maximal angle of deflection resulting from such heuristic calculations is only half the value given by general relativity.[59] Important observable examples involve the light of stars or distant quasars being deflected as it passes the Sun.[60]

Closely related to light deflection is the gravitational time delay (or Shapiro effect): light signals take longer to move through a gravitational field than they would in the absence of that field. There have been numerous successful tests of this prediction.[61] In the parameterized post-Newtonian formalism (PPN), measurements of both the deflection of light and the gravitational time delay determine a parameter called γ, which encodes the influence of gravity on the geometry of space.[62]

Gravitational waves

Main article: Gravitational waves

One of several analogies between weak-field gravity and electromagnetism is that, analogous to electromagnetic waves, there are gravitational waves: spacetime ripples which propagate at the speed of light.[63] The simplest variety can be visualized by its action on a ring of freely floating particles (first image to the right). A sine wave propagating through such a ring towards the reader distorts the ring in a characteristic, rhythmic fashion (second image to the right).[64] Such linearized gravitational waves are important when it comes to describing the exceedingly weak waves that are expected to arrive here on Earth from far-off cosmic events, which typically result in distances increasing and decreasing by 10 − 21 or less. Data analysis methods routinely make use of the fact that these linearized waves can be Fourier decomposed.[65]

Einstein's equations are non-linear, so there is no linear superposition for arbitrarily strong gravitational waves, making their description a difficult task. There are some exact solutions describing gravitational waves, for instance a wave train traveling through empty space[66] or so-called Gowdy universes, varieties of an expanding cosmos filled with gravitational waves.[67] But for gravitational waves produced in astrophysically relevant situations, such as the merger of two black holes, numerical methods are presently the only way to construct appropriate models.[68]

Orbital effects and the relativity of direction

Main article: Kepler problem in general relativity

General relativity differs from classical mechanics in a number of predictions concerning orbiting bodies. It predicts the relativistic apside shifts, as well as orbital decay caused by the emission of gravitational waves and effects related to the relativity of direction.

Precession of apsides

In general relativity, the apsides of any orbit (the point of the orbiting body's closest approach to the system's center of mass) will precess—the orbit is not an ellipse, but akin to an ellipse that rotates on its focus, resulting in a rosette-like shape (see image). Einstein first derived this result by using an approximate metric representing the Newtonian limit and treating the orbiting body as a test particle. For him, the fact that his theory gave a straightforward explanation of the anomalous perihelion shift of the planet Mercury, discovered earlier by Urbain Le Verrier in 1859, was important evidence that he had at last identified the correct form of the gravitational field equations.[69]

The effect can also be derived by using either the exact Schwarzschild metric (describing spacetime around a spherical mass)[70] or the much more general post-Newtonian formalism.[71] It is due to the influence of gravity on the geometry of space and to the contribution of self energy to a body's gravity (encoded in the nonlinearity of Einstein's equations).[72] Relativistic precession has been observed for all planets that allow for accurate precession measurements (Mercury, Venus and the Earth),[73] as well as in binary pulsar systems, where it is larger by five orders of magnitude.[74]

Orbital decay

According to general relativity, a binary system will emit gravitational waves, thereby losing energy. Due to this loss, the distance between the two orbiting bodies decreases, and so does their orbital period. Within the solar system or for ordinary double stars, the effect is too small to be observable. Not so for a close binary pulsar, a system of two orbiting neutron stars, one of which is a pulsar: from the pulsar, observers on Earth receive a regular series of radio pulses that can serve as a highly accurate clock, which allows precise measurements of the orbital period. Since the neutron stars are very compact, significant amounts of energy are emitted in the form of gravitational radiation.[76]

The first observation of a decrease in orbital period due to the emission of gravitational waves was made by Hulse and Taylor, using the binary pulsar PSR1913+16 they had discovered in 1974. It amounts to the first indirect detection of gravitational waves, rewarded with the Nobel Prize in physics in 1993.[77] Since then, several other binary pulsars have been found, in particular the double pulsar PSR J0737-3039, in which both stars are pulsars.[78]

Geodetic precession and frame-dragging

Main articles: Geodetic precession and Frame dragging

Several relativistic effects are directly related to the relativity of direction.[79] One is geodetic precession: the axis direction of a gyroscope in free fall in curved spacetime will change when compared, for instance, with the direction of light received from distant stars—even though such a gyroscope represents the way of keeping a direction as stable as possible ("parallel transport").[80] For the Moon-Earth-system, this effect has been measured with the help of lunar laser ranging.[81] More recently, it has been measured for test masses aboard the satellite Gravity Probe B to a precision of better than 1 percent.[82]

Near a rotating mass, there are so-called gravitomagnetic or frame-dragging effects. A distant observer will determine that objects close to the mass get "dragged around". This is most extreme for rotating black holes where, for any object entering a zone known as the ergosphere, rotation is inevitable.[83] Such effects can again be tested through their influence on the orientation of gyroscopes in free fall.[84] Somewhat controversial tests have been performed using the LAGEOS satellites, confirming the relativistic prediction.[85] A precision measurement is the main aim of the Gravity Probe B mission, with the results expected in September 2008.[86]

Astrophysical applications

Gravitational lensing

Main article: Gravitational lensing

The deflection of light by gravity can lead to an intriguing phenomenon. If there is a massive object between the observer and a distant target object, with the mass and relative distances just right, the observer will see multiple distorted images of the target. This and similar effects are known as gravitational lensing.[87] Depending on the configuration, scale, and mass distribution, there can two or more images, a bright ring known as an Einstein ring, or partial rings called arcs.[88] The earliest example was discovered in 1979;[89] since then, more than a hundred gravitational lenses have been observed.[90] Multiple images too close to be resolved can still lead to a measurable effect, an overall brightening of a given star or other point-like object; a number of such "microlensing events" has been observed, as well.[91]

Gravitational lensing has developed into a tool of observational astronomy. It is used to detect the presence and distribution of dark matter, provide a "natural telescope" for observing distant galaxies, and to obtain an independent estimate of the Hubble constant. Statistical evaluations of lensing data provide valuable insight into the structural evolution of galaxies.[92]

Einstein cross: four images of the same astronomical object, produced by a gravitational lens

Gravitational wave astronomy

Main article: Gravitational waves

Observations of binary pulsars provide strong indirect evidence for the existence of gravitational waves (see the section on Orbital decay, above). However, gravitational waves reaching us from the depths of the cosmos have not been detected directly, this being one of the major goals of current relativity-related research.[93] To this end, a number of land-based gravitational wave detectors are currently in operation, most notably the interferometric detectors GEO 600, LIGO (three detectors), TAMA 300 and VIRGO.[94] A joint US-European space-based detector, LISA, is currently under development,[95] with a precursor mission (LISA Pathfinder) due for launch in late 2009.[96]

Gravitational waves promise to yield information about astronomical objects that is inaccessible by observations using electromagnetic radiation:[97] Terrestrial detectors are expected to yield new information about inspiral phase and mergers of binary stellar mass black holes and binaries consisting of one such black hole and a neutron star (of interest as a candidate mechanism for gamma ray bursts). They could also detect signals from core-collapse supernovae and from periodic sources such as rotating neutron stars with small deformation. If there is truth to speculation about certain kinds of phase transitions or kink bursts from long cosmic strings in the very early universe (at cosmic times around 10 − 25 seconds) these could also be detectable.[98] Space-based detectors like LISA should detect objects such as binaries consisting of two White Dwarfs, and AM CVn stars (a White Dwarf accreting matter from its binary partner, a low-mass helium star), and also observe the mergers of supermassive black holes and the inspiral of smaller objects (between one and a thousand solar masses) into such black holes. LISA should also be able to listen to the same kind of sources from the early universe as ground-based detectors, but at even lower frequencies and with greatly increased sensitivity.[99]

Black holes and other compact objects

Main article: Black hole

Whenever an object becomes sufficiently compact, general relativity predicts the formation of a black hole: a region of space from which nothing, not even light, can escape. In the currently accepted models of stellar evolution, neutron stars with around 1.4 solar mass and so-called stellar black holes with a few to a few dozen solar masses are thought to be the final state for the evolution of massive stars.[100] Supermassive black holes with a few million to a few billion solar masses are considered the rule rather than the exception in the centers of galaxies,[101] and their presence is thought to have played an important role in the formation of galaxies and larger cosmic structures.[102]

Astronomically, the most important property of compact objects is that they provide a superbly efficient mechanism for converting gravitational energy into electromagnetic radiation.[103] Accretion, the falling of dust or gaseous matter onto stellar or supermassive black holes, is thought to be responsible for some spectacularly luminous astronomical objects, notably diverse kinds of active galactic nuclei on galactic scales and stellar-size objects such as Microquasars.[104] In particular, accretion can lead to relativistic jets, focused beams of highly energetic particles that are being flung into space at almost light speed.[105] Interestingly, to a distant observer, some of these jets even appear to move faster than light; this, however, can be explained as an optical illusion that does not violate the tenets of relativity.[106] General relativity plays a central role in modelling all these phenomena,[107] relativistic lensing effects being thought to play a role for the signals received from X-ray pulsars.[108]

Limits on compactness from the observation of accretion-driven phenomena ("Eddington luminosity"),[109] observations of stellar dynamics in the center of our own Milky Way galaxy,[110] and indications that at least some of the compact objects in question appear to have no solid surface[111] provide strong indirect evidence for the existence of black holes. Direct evidence, such as observing the "shadow" of the Milky Way galaxy's central black hole horizon, is eagerly sought for.[112]

Black holes are also sought-after targets in the search for gravitational waves (see the section Gravitational waves, above): merging black hole binaries should lead to some of the strongest gravitational wave signals reaching detectors here on Earth, and reliable simulations of such mergers are one of the main goals of current research in numerical relativity.[113] The phase directly before the merger ("chirp") could be used as a "standard candle" to deduce the distance to the merger events, and hence as a probe of cosmic expansion at large distances.[114] The gravitational waves produced as a stellar black hole plunges into a supermassive one should serve as a probe of the supermassive black hole's geometry.[115]

Cosmology

Main article: Physical cosmology

Each solution of Einstein's equations describes a whole universe, so it should come as no surprise that there are solutions that provide useful models for cosmology, the study of the universe as a whole. The current models are based on an extension of the original form of Einstein's equations which include the cosmological constant Λ, an additional term that has an important influence on the large-scale dynamics of the cosmos,

where gab is the spacetime metric.[116]

On the basis of isotropic and homogeneous solutions of these enhanced equations, the so-called Friedmann-Lemaître-Robertson-Walker solutions,[117] are built the models of modern cosmology in which the universe has evolved over the past 14 billion years from a hot, early Big Bang phase.[118] Once a small number of parameters (for example the universe's mean matter density) have been fixed by astronomical observation,[119] further observational data can be used to put the models to the test: successful predictions include the initial abundance of chemical elements formed in a period of primordial nucleosynthesis,[120] which is in good agreement with astronomical observations,[121] and the large-scale distribution of galaxies.[122] Further important evidence is provided by the existence and properties of a "thermal echo" from the early cosmos, the cosmic background radiation, which contains information about the cosmological parameters, the universes matter and energy content, and the seeds of today's large scale structure.[123] Future measurements could also reveal evidence about gravitational waves in the early universe.[124]

The status of the resulting models is mixed. On the one hand, the standard models of cosmology have been very successful: to date, they have passed all observational tests,[125] and they have proven a sound basis to explaining the evolution of the universe's large-scale structure.[126] On the other hand, there are a number of important open questions. The determination of cosmological parameters (in line with other astronomical observations[127]) suggests that about 90 percent of all matter in the universe is in the form of so-called dark matter, which has mass (and hence gravitational influence), but does not interact electromagnetically (and hence cannot be observed directly). There is currently no generally accepted description of this new kind of matter within the framework of particle physics[128] or otherwise.[129] A similar open question is that of dark energy. Observational evidence from redshift surveys of distant supernovae and measurements of the cosmic background radiation show that the evolution of our universe is significantly influenced by a cosmological constant resulting in an acceleration of cosmic expansion or, equivalently, by a form of energy with an unusual equation of state, namely dark energy.[130] The nature of this new form of energy remains unclear.[131]

A number of further problems of the classical cosmological models (such as "why is the cosmic background radiation so highly homogeneous")[132] have led to the introduction of an additional phase of strongly accelerated expansion at cosmic times of around 10 − 33 seconds, known as an inflationary phase.[133] While recent measurements of the cosmic background radiation have resulted in first evidence for this scenario,[134] problems remain. There is a bewildering variety of possible inflationary scenarios not restricted by current observations.[135] Also, the question remains what happened in the earliest universe, close to where the classical models predict the big bang singularity. An authoritative answer would require a complete theory of quantum gravity, which does not exist at the moment[136] (cf. the section on quantum gravity, below).

Advanced concepts

Causal structure and global geometry

Main article: Causal structure

In general relativity, no material body can catch up with or over take a light pulse; no influence from an event A can reach any other location before light sent out at A does so. Hence, an exploration of all light worldlines (null geodesics) yields key information about the spacetime's causal structure. This structure can be displayed using Penrose-Carter diagrams in which infinitely large regions of space and infinite time intervals are shrunk ("compactified") so as to fit onto a finite map, while light still travels along diagonals as in standard spacetime diagrams.[137]

Aware of the importance of causal structure, Roger Penrose and others developed important techniques that are now termed global geometry. In global geometry, the object of study is not one particular solution (or family of solutions) to Einstein's equations. Rather, relations that hold true for all geodesics, such as the Raychaudhuri equation, are utilized in conjunction with non-specific assumptions about the nature of matter (usually in the form of so-called energy conditions) to derive general results.[138]

Horizons

Main articles: Horizon (general relativity), No hair theorem, and Black hole mechanics

One of the most striking conclusions that can be drawn from studies of global geometry is the existence of boundaries called horizons, which demarcate one spacetime region from the rest of the spacetime. The best-known examples are black holes: if mass is compressed into a sufficiently compact region of space (as specified in the hoop conjecture, the relevant length scale being the Schwarzschild radius[139]), spacetime is partitioned into an inside and an outside world: no light from the inside can escape to the outside, and since, in general relativity, no object can overtake a light pulse, all inside matter is imprisoned as well. However, matter and radiation may cross the horizon into the black hole—clearly showing that horizons are not physical barriers. The resulting object is known as a black hole, and the surface in question as the black hole's horizon.[140]

Initial black hole studies relied on simplified models obtained from explicit solutions of Einstein's equation, notably the spherically-symmetric Schwarzschild solution (used to describe a static black hole) and the axisymmetric Kerr solution (used to describe a rotating, stationary black hole, and introducing interesting features such as the ergosphere). Subsequent studies using global geometry have revealed more general properties of black holes: in the long run, they are rather simple objects characterized by eleven parameters specifying energy, linear momentum, angular momentum, location at a specified time and electric charge. This is stated by the black hole uniqueness theorems: "black holes have no hair", that is, no distinguishing marks like the hairstyles of humans: irrespective of the complexity of a gravitating object collapsing to form a black hole, the object that results (having emitted gravitational waves) is very simple.[141]

Even more remarkably, there is a general set of laws known as black hole mechanics, which is analogous to the laws of thermodynamics. For example, by the second law of black hole mechanics, the area of the event horizon of a general black hole will never decrease with time, analogous to the entropy of a thermodynamic system. This limits the energy that can be extracted from a rotating black hole (e.g. by the Penrose process).[142] There is strong evidence that the laws of black hole mechanics are, in fact, a special case of the laws of thermodynamics, and that the black hole area does indeed denote its entropy:[143] semi-classical calculations indicate that black holes do emit thermal radiation, with the surface gravity playing the role of temperature in Planck's law. This radiation is known as Hawking radiation (cf. the quantum theory section, below).[144]

Horizons also play a role for other kinds of solutions. In an expanding universe, some regions of the past can be unobservable ("particle horizon"), and some regions of the future cannot be influenced (event horizon). In both cases, the location of the horizon in spacetime depends on the event in question.[145] Even in flat Minkowski space, when described by an accelerated observer (Rindler space), there will be horizons[146] (associated with a semi-classical radiation known as Unruh radiation).[147]

Singularities

Main article: Spacetime singularity

Another general—and quite disturbing—feature of general relativity is the appearance of spacetime boundaries known as singularities. Ordinary spacetime can be explored by following up on all possible ways that light and particles in free fall can travel (that is, all timelike and lightlike geodesics). But there are spacetimes which fulfill all the requirements of Einstein's theory, yet have "ragged edges"—regions where the paths of light and falling particles come to an abrupt end and geometry becomes ill-defined. By definition, these are spacetime singularities. In more interesting cases, the geometrical quantities characterizing spacetime curvature (e.g. the Ricci scalar) take on infinite values at such "curvature singularities".[148] Well-known examples of spacetimes with future singularities—where worldlines end—are the Schwarzschild solution, which describes a singularity inside an eternal static black hole,[149] or the Kerr solution with its ring-shaped singularity inside an eternal rotating black hole.[150] The Friedmann-Lemaître-Robertson-Walker solutions, and other spacetimes describing universes, have past singularities on which worldlines begin, namely big bang singularities, and some have future singularities (big crunch) as well.[151]

From these examples, all highly symmetric and thus simplified, one might think the occurrence of singularities to be a consequence of idealization. The famous singularity theorems proved using the methods of global geometry say otherwise: singularities are a generic feature of general relativity, and unavoidable once the collapse of an object with realistic matter properties has proceeded beyond a certain stage[152] and also at the beginning of a wide class of expanding universes.[153] However, these theorems say very little about the properties of singularities, and much of current research is devoted to characterizing these entities' generic structure (hypothesized e.g. by the so-called BKL conjecture).[154] The cosmic censorship hypothesis states that all realistic future singularities (no perfect symmetries, matter with realistic properties) are safely hidden away behind a horizon, and thus invisible to all distant observers. While no formal proof yet exists, numerical simulations offer supporting evidence of its validity.[155]

Evolution equations

Main article: Initial value formulation (general relativity)

Each solution of Einstein's equation encompasses the whole history of a universe—it is not just some snapshot of how things are, but a whole spacetime: a statement encompassing the state of matter and geometry everywhere and at every moment in that particular universe. By this token, Einstein's theory appears to be different from most other physical theories, which specify evolution equations for physical systems: if the system is in a given state at some given moment, the laws of physics allow you to extrapolate its past or future. For Einstein's equations, there appear to be subtle differences compared with other fields, for example, they are self-interacting (that is, non-linear even in the absence of other fields, and they have no fixed background structure—the stage itself evolves as the cosmic drama is played out).[156]

Nevertheless, in order to understand Einstein's equations as partial differential equations, it is crucial to re-formulate them in a way that describes the evolution of the universe over time. This is achieved by so-called "3+1" formulations, where spacetime is split into three space dimensions and one time dimension, such as the ADM formalism.[157] These decompositions show that the spacetime evolution equations of general relativity are indeed well-behaved, meaning that solutions always exist and are uniquely defined (once suitable initial conditions are specified).[158] Formulations like this are also the basis of numerical relativity: attempts to simulate the evolution of relativistic spacetimes (notably merging black holes or gravitational collapse) using computers.[159]

Global and quasi-local quantities

Main article: Mass in general relativity

The notion of evolution equations is intimately tied in with another aspect of general relativistic physics. In Einstein's theory, it turns out to be impossible to find a general definition for a seemingly simple property such as a system's total mass (or energy). The main reason for this is that the gravitational field—like any physical field—must be ascribed a certain energy. However, it is fundamentally impossible to localize that energy.[160]

Nevertheless, there are possibilities to define a system's total mass, either using a hypothetical "infinitely distant observer" (ADM mass)[161] or suitable symmetries (Komar mass).[162] If one excludes from the system's total mass the energy being carried away to infinity by gravitational waves, the result is the so-called Bondi mass at null infinity.[163] Just as in classical physics, it can be shown that these masses are positive.[164] Analogous global definitions exist for momentum and angular momentum.[165] In addition, there have been a number of attempts to define quasi-local quantities, such as the mass of an isolated system formulated using only quantities defined within a finite region of space containing that system. The hope is to obtain a quantity useful for general statements about isolated systems, such as a more precise formulation of the hoop conjecture.[166]

Relationship with quantum theory

Along with general relativity, quantum theory, the basis of our understanding of matter from elementary particles to solid state physics, is considered one of the two pillars of modern physics.[167] However, it is still an open question of how the concepts of quantum theory can be reconciled with those of general relativity.

Quantum field theory in curved spacetime

Main article: Quantum field theory in curved spacetime

Ordinary quantum field theories, which form the basis of modern elementary particle physics, are defined in flat Minkowski space, which is an excellent approximation when it comes to describing the behavior of microscopic particles in weak gravitational fields like those found on Earth.[168] In order to describe situations in which gravity is strong enough to influence (quantum) matter, yet not strong enough to require quantization itself, physicists have formulated quantum field theories in curved spacetime. These theories rely on classical general relativity to describe a curved background spacetime, and define a generalized quantum field theory to describe the behavior of quantum matter within that spacetime.[169] Using this formalism, it can be shown that black holes emit a blackbody spectrum of particles known as Hawking radiation, leading to the possibility that the evaporate over time.[170] As briefly mentioned above, this radiation plays an important role for the thermodynamics of black holes.[171]

Quantum gravity

Main article: Quantum gravity

See also: String theory and Loop quantum gravity

The demand for consistency between a quantum description of matter and a geometric description of spacetime,[172] as well as the appearance of singularities (where curvature length scales become microscopic), indicate the need for a full theory of quantum gravity: for an adequate description of the interior of black holes, and of the very early universe, a theory is required in which gravity and the associated geometry of spacetime are described in the language of quantum physics.[173] Despite major efforts, no complete and consistent theory of quantum gravity is currently known, even though a number of promising candidates exists.[174]

Attempts to generalize ordinary quantum field theories, used in elementary particle physics to describe fundamental interactions, so as to include gravity have led to serious problems. At low energies, this approach proves successful, in that it results in an acceptable effective (quantum) field theory of gravity.[175] At very high energies, however, the result are models devoid of all predictive power ("non-renormalizability").[176]

One attempt to overcome these limitations is to formulate a quantum theory not of point particles, but of minute one-dimensional extended objects: string theory.[177] The theory promises to be a unified description of all particles and interactions, including gravity;[178] the price to pay are unusual features such as six extra dimensions of space in addition to the usual three.[179] In what is called the second superstring revolution, it was conjectured that both string theory and a unification of general relativity and supersymmetry known as supergravity[180] form part of a hypothesized eleven-dimensional model known as M-theory, which would constitute a uniquely defined and consistent theory of quantum gravity.[181]

Another approach starts with the canonical quantization procedures of quantum theory. Using the initial-value-formulation of general relativity (cf. the section on evolution equations, above), the result is an analogue of the Schrödinger equation: the Wheeler-deWitt equation which, regrettably, turns out to be ill-defined.[182] However, with the introduction of what are now known as Ashtekar variables,[183] this leads to a promising model known as Loop quantum gravity. Space is represented by a network structure called a spin network, evolving over time in discrete steps.[184]

Depending on which features of general relativity and quantum theory are accepted unchanged, and on what level changes are introduced,[185] there are numerous other attempts to arrive at a viable theory of quantum gravity, some example being dynamical triangulations,[186] causal sets,[187] twistor models[188] or the path-integral based models of quantum cosmology.[189]

All candidate theories still have major formal and conceptual problems to overcome. They also face the common problem that, as yet, there is no way to put quantum gravity predictions to experimental tests, although there is hope for this to change as future data from cosmological observations and particle physics experiments becomes available.[190]

Current status

General relativity has emerged as a highly successful model of gravitation and cosmology, which has so far passed every unambiguous observational and experimental test. Even so, there are strong indications the theory is incomplete.[191] The problem of quantum gravity and the question of the reality of spacetime singularities remain open.[192] Observational data that is taken as evidence for dark energy and dark matter could indicate the need for new physics,[193] and while the so-called Pioneer anomaly might yet admit of a conventional explanation, it, too, could be a harbinger of new physics.[194] Even taken as is, general relativity is rich with possibilities for further exploration. Mathematical relativists seek to understand the nature of singularities and the fundamental properties of Einstein's equations,[195] and increasingly powerful computer simulations (such as those describing merging black holes) are run.[196] The race for the first direct detection of gravitational waves continues apace,[197] in the hope of creating opportunities to test the theory beyond the limited approximations it has been tested so far even in the binary pulsar measurements.[198] More than ninety years after its publication, general relativity remains a highly active area of research.[199]

See also

* Contributors to general relativity

* Einstein-Hilbert action

* General relativity resources, an annotated reading list giving bibliographic information on some of the most cited resources

* Introduction to mathematics of general relativity

* Timeline of gravitational physics and relativity

Notes

References

Links