In four dimensional geometry, the cantitruncated 5-cell is a uniform polychoron. It is composed of 60 vertices, 120 edges, 80 faces, and 20 cells. The cells are: 5 truncated octahedra, 10 triangular prisms, and 5 truncated tetrahedra. Each vertex is surrounded by 2 truncated octahedra, one triangular prism, and one truncated tetrahedron.

Alternative names

* Cantitruncated pentachoron
* Cantitruncated 4-simplex
* Great prismatodispentachoron
* Truncated dispentachoron
* Grip (Jonathan Bowers: for great rhombated pentachoron)

Images

The Cartesian coordinates of an origin-centered cantitruncated 5-cell having edge length 2 are:

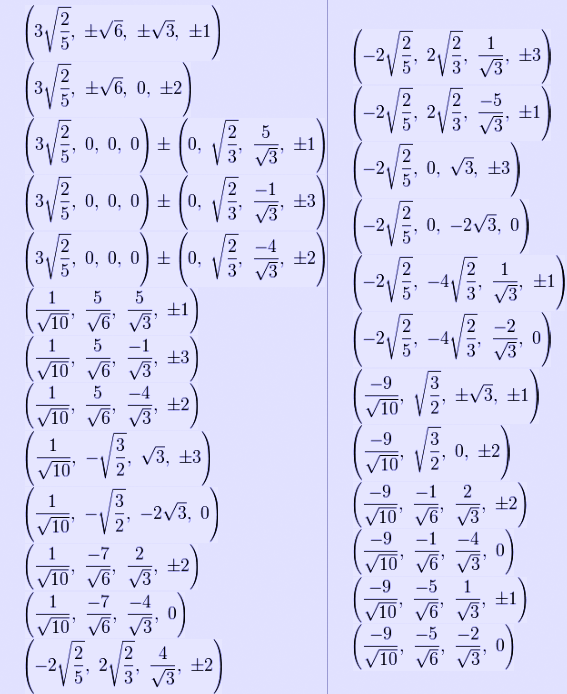
These vertices can be more simply constructed on a hyperplane in 5-space, as the permutations of:

(0,0,1,2,3)

This construction is from the positive orthant facet of the cantitruncated pentacross.

Related uniform polychora

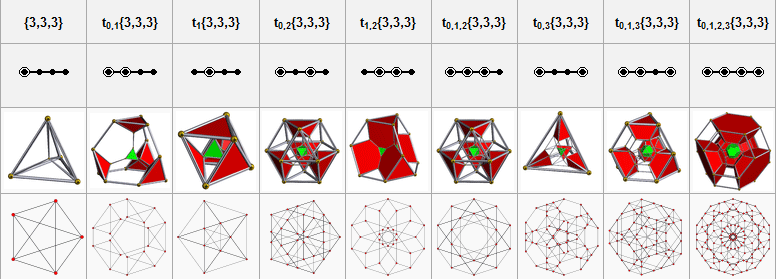
The cantitruncated pentachoron is one of 9 uniform polychora constructed from the [3,3,3] Coxeter group.

Name	5-cell	truncated 5-cell	rectified 5-cell	cantellated 5-cell	bitruncated 5-cell	cantitruncated 5-cell	runcinated 5-cell	runcitruncated 5-cell	omnitruncated 5-cell
Schläfli symbol
Coxeter-Dynkin diagram
Schlegel diagram
Coxeter plane projection

Images: Robert Webb's Great Stella software